Horn Torus Model
 
In the following we want to go a bit further into the model, but to emphasize it again: the horn torus model is neither a mathematical nor a physical theory, it is not science at all, it is rather a symbolic, analogical representation, using allegoric visualisations of fundamental physical entities, playfully aiming for different interpretations of fundamental processes, for an exciting creation of novel insights and for an amazing enhancement of existing explanations. Don’t expect a stringent and structured scientific treatise, but note instead, that all statements remain on an ontological, epistemological, philosophical level. *
 
The horn torus model is an attempt and a proposal to describe foundations of our 'real world' in an assumed manifestation as unconscious universe with no properties others than mathematical. Constituents aren't space, time and matter, but only numbers in their simplest form: natural numbers, as complex integers first, later - as required - easily extendable to quaternions, octonions, sedenions, again with integer parts each. Nested horn tori, dynamically interlaced into one another, illustrate as eidetic geometric analogue, that numbers quasi are able to interact with one another and - e.g. by mutual 'annihilation' - to transform their initial continuum into discrete structures by a simple pattern generating process, leading to intricate results, which are interpretable as entities or 'coordinates' of a dynamic but timeless space with striking analogies to our 'real world’.
 
We indeed possess mathematical descriptions for all realized physical processes and apply them very successfully, without any doubt, but no actual accepted model of nature describes all in one big idea, no one comprises all aspects of experienceable ‘reality’, and furthermore - most important - there is a lack of appropriate images which illustrate objects and processes in such a way that they match the human thought structure and mental capability adequately.
 
Here starts the relevance of horn tori. The associated model tries to fill the lack of imagery, what most of us need for a good understanding. Dynamization of horn tori, illustrated in an easily intelligible pictorial way, generates multifarious and sophisticated new properties, and there will open a completely new mathematical world which bears the possibility to see and describe the fundaments of our ‘real world’ in a quite different and potentially most comprehensive way. Dynamic of space is the magic word. Not in the sense of geometrodynamic, where our familiar three- or more-dimensional space somehow is distorted dynamically. The space, we head for with the horn tori, is not spanned by dimensions, it is neither a Euclidean nor non-Euclidean space, nor any vector space, not even in the sense of the abstract Hilbert space - it is spanned instead by continuously and dynamically changing coordinates.
 
We dynamize the primarily static figure horn torus - I repeat that again and again, to emphasise the underlying principle - by introducing rotation around the main symmetry axis and revolution of the torus bulge around itself, detect the then emerging trajectories on the surface, observe their paths, which depend on the ratio of turns, let the horn torus change its size according to the ‘unrolled distance’ by the revolution, what again changes the mentioned ratio and the trajectories, then interlace different horn tori by nesting them into one another with common centre, interpret the superposition or mutual interference of trajectories as particle interaction, finally abstract from the three-dimensional space of imagination to the effect that we renunciate dimensions at all, which in common view span a physical space as container for physical objects, and - as quintessence of all previous mental efforts - detect that our new space is ‘dimensionless’.
 
That all sounds extremely intricate and artificial, and to realise, that the three-dimensional space (likewise all linear vector spaces!) shall be a man-made construct and not an a priori existing entity, seems to be difficult to place within the scientific community. But an open-minded unbiased readiness for broad familiarization with the allegoric images and processes, leading to a far-reaching abstraction from most traditional ideas, soon enables you to revel in new interpretations, perceptions, cognitions, and lots of aha experiences.
 
The dynamic horn torus model highlights and perhaps reveals big mysteries in our comprehension of ‘reality’, at least in an allegorical, descriptive sense for issues concerning intrinsicality of time, space and physical objects, continuum vs. discrete nature of space, metrisation of space, origin and cause for quantization, minimum values, constancy and maximum value of lightspeed, non-locality of quantum processes, entanglement, arrow of time, determinism, causality, ...
 
In our intellectual game we reduce the many (too many!) necessary components of the standard model to one single principle, which underlies all physical objects and processes as a unified mathematical structure or rule, by introducing graphically describable and imaginable all-embracing fundamental entities. But again: I want to emphasize, that the horn torus model is neither physics nor mathematics, and least of all it is esotericism or anything like ‘holy’ or ‘sacred’ geometry! It is a pure intellectual game to realize generally, that fundamental entities as representation of physical objects, processes, elementary particles, and space are imaginable and constructable.
 
Origin of the idea (in 1988) was the endeavour to find an analogously figurative visualisation of complex numbers, different from the well-known static Riemann sphere. Target was to illustrate dynamic physical processes which very often are described mathematically by means of complex numbers - be it in electrodynamics, relativity or in quantum mechanics. As proposal then emerged that the real part shall be represented by the rotation of a horn torus around the main symmetry axis, the imaginary part by the revolution of the torus bulge around itself, measured in degrees of rotation respectively revolution, not as localisation on the horn torus surface. The dynamic figure replaces the Riemann sphere, and values of complex numbers thus are represented and visualised dynamically.*
 
Main principle of the dynamic horn torus is that it rolls along an imaginary axis i·t with constant ‘speed’ c and changes its size according to the unrolled ‘distance’ on this axis. Without rotation around the axis, the difference of horn torus longitudes before and after a motion corresponds to the unrolled distance during ‘locomotion’, shown in link [3]. Circumferential speed is constant, but angular velocity of revolution depends on size: these latter quantities are inversely proportional. When we now add rotation, the situation gets more intricate: the place of the unrolling longitudes undertakes a trajectory, which figuratively unrolls without ‘slippage’ on the axis.
 
These trajectories, ‘unrolling lines’ or cycloids show a particular pattern on the horn torus surface, according to the ratio of revolution and rotation, more precisely, to the ratio of their angular velocities. Here, in fig. 2, we show some trajectories for different ratios in a perspective view:

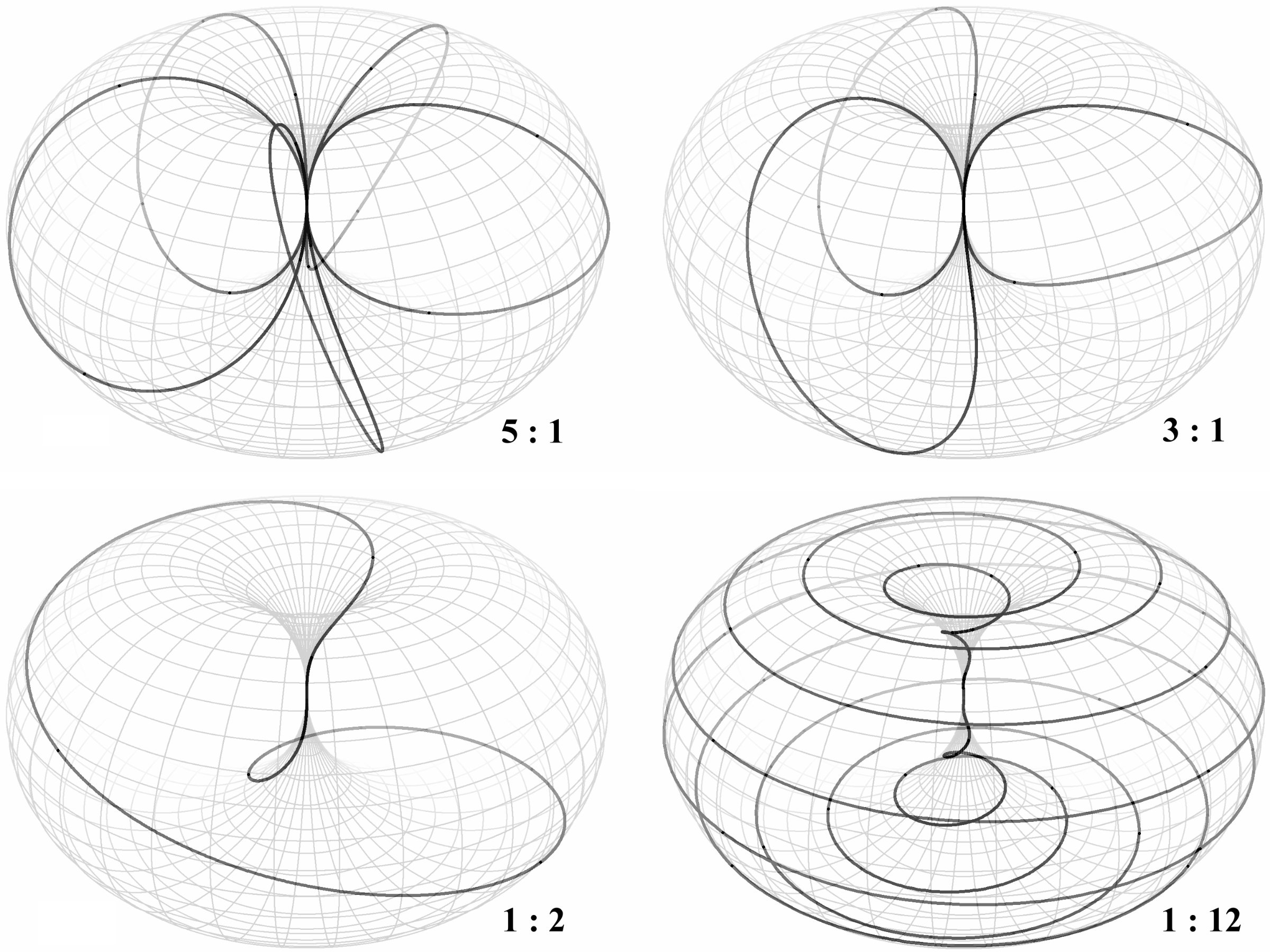
The examples in fig. 2 show trajectories for rational ratios (revolution : rotation). For ratios ≥ 1 they loop (repeat) after one rotation, for ratios ≤ 1 after one revolution. I call them resonances or Lissajous figures on horn torus surfaces. During unrolling along the axis, a horn torus changes its size and therefore passes all possible ratios and all resonances as well. For small horn tori we see many revolutions per rotation and for big horn tori many rotations per revolution. A kind of mathematical mirror is the ratio 1:1, and in the physical interpretations it will appear to have a very special meaning. The related figurative object (fig. 3) is called ‘standard dynamic horn torus’.

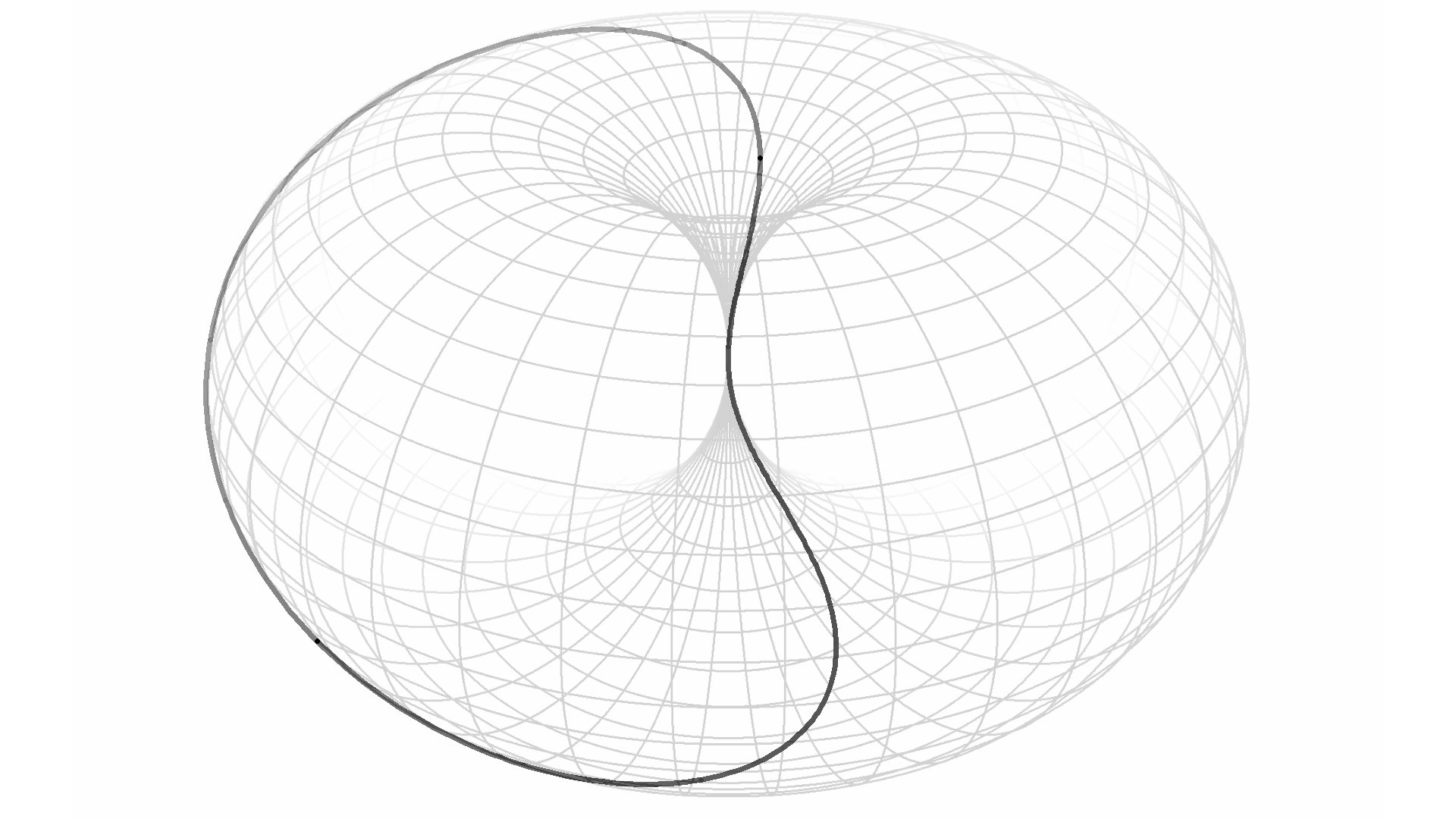
To anticipate later considerations: the standard dynamic horn torus already symbolises some of the most fundamental quantities. For this unit horn torus, we determine that one revolution represents Planck time t_P and the circumference of a longitude (meridian) Planck length l_P (in a different interpretation: length of the 1:1-trajectory), while the circumferential speed of revolution is light speed c, and one rotation represents Planck’s reduced constant ħ.
 
The unit horn torus divides the set of all (infinitely many) possible horn tori into two parts. Those with ratio revolution : rotation > 1 have a smaller (down to infinitesimal) size, compared with this unit, and we see trajectories with more than one loop around the torus bulge per one rotation, while tori with ratio < 1 are bigger (up to infinitely large) with more than one ‘spiral’ around the rotational axis per one revolution of the bulge (compare examples in fig. 2).
 
The complete set comprises all horn tori, all sizes, and all ratios (but note, size and ratio are not independent, they are inversely proportional!), and we designate it as ‘fundamental entity’. A particular horn torus occurs exactly once in the set.
 
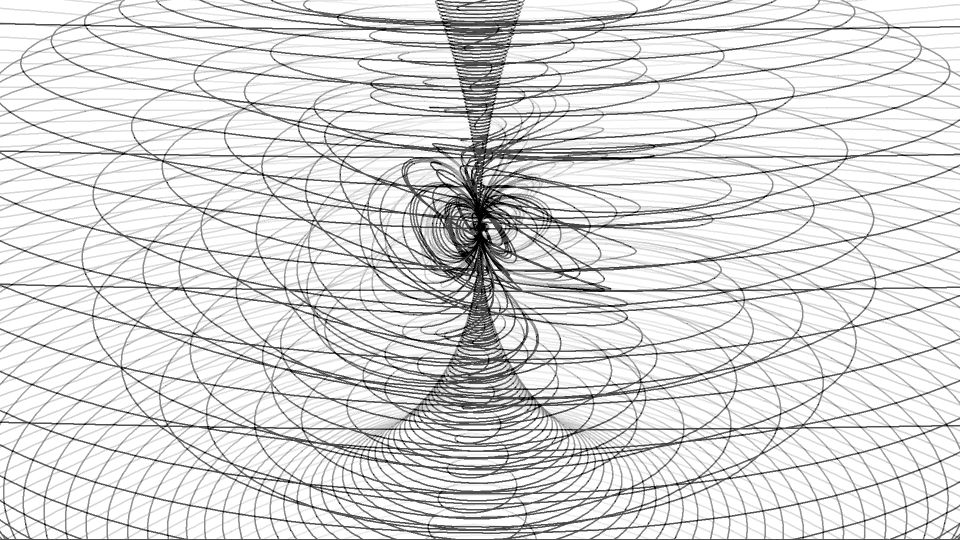
To give an impression of the complexity, we now draw a simplified graph of this entity, represented by the ‘unrolling line’ in dependence of an increasing r, selected between ratios v(φ) : v(ω) = 10 : 1 and v(φ) : v(ω) = 1 : 1000, but not to scale and using rather rough differentials dr for the single steps (otherwise the lines would lay very densely). r is horn torus radius, v angular velocity, φ revolution, ω rotation. See again animation in link [2].

The related parametric form must be established for both parts of the entity separately in the following way (setting r = 1 for ratio v(φ) : v(ω) = 1 : 1)
 
For case r > 1, r and ω increase with φ, starting with φ₁ = 2π,
 
and according to r = φ / 2π (↝ r₁ = 1), ω = r·φ = φ² / 2π (↝ ω₁ = 2π), we have
 
x = (1 – cos(φ))·cos(φ² / 2π)·φ/2π
 
y = (1 – cos(φ))· sin(φ² / 2π)·φ/2π
 
z = sin(φ) · φ/2π
 
For case r < 1, 1/r and φ increase with ω, starting with ω₁ = 2π,
 
and according to 1/r = ω / 2π (↝ r₁ = 1), φ = ω / r = ω² / 2π (↝ φ₁ = 2π), we have
 
x = (1 − cos(ω² / 2π))·cos(ω)·2π/ω
 
y = (1 − cos(ω² / 2π))· sin(ω)·2π/ω
 
z = sin(ω² / 2π)· 2π/ω
 
The parametric forms show mirrored conditions, so, as already mentioned, the unit standard dynamic horn torus indeed is a kind of mirror within the new mathematical world, and likewise, as physical interpretation, it separates the big ‘outer’ world, consisting of electrons and photons from the small ‘inner’ atomic and nuclear realm.
 
Next step should be, that we interlace entities into one another, maintaining a common point for all, namely the point S (S for symmetry or singularity), the centre of all nested horn tori. But alone the complexity of one single entity indicates that this endeavour appears to have no prospect of success, when we apply common mathematics only. But it could be worse. For our aim, to get an idea of the model, it is enough to understand the principle of nesting two or a few horn tori into one another. As first impression here two (fig. 5, animated in link [5]) and twelve (fig. 6) nested horn tori:
 

 
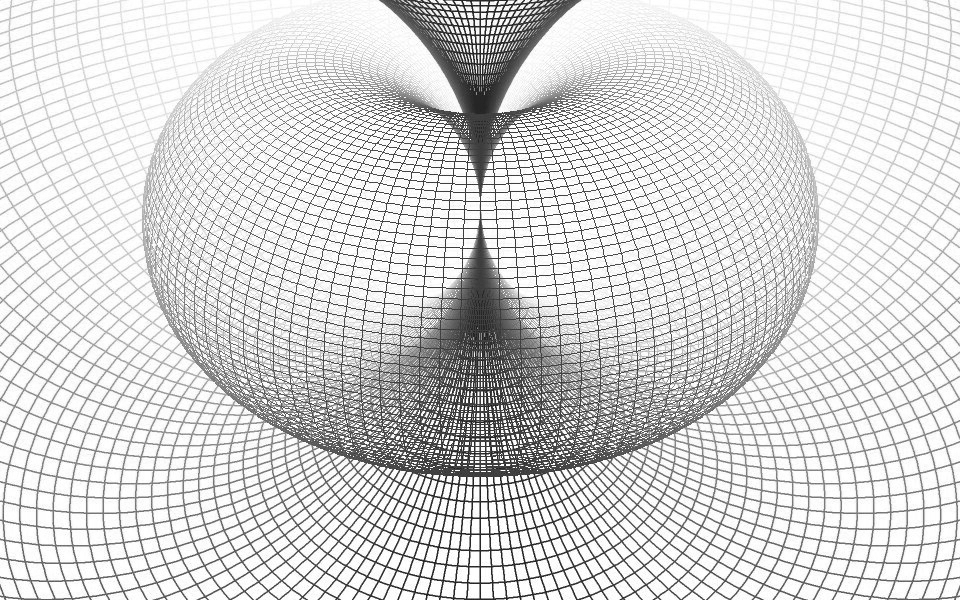
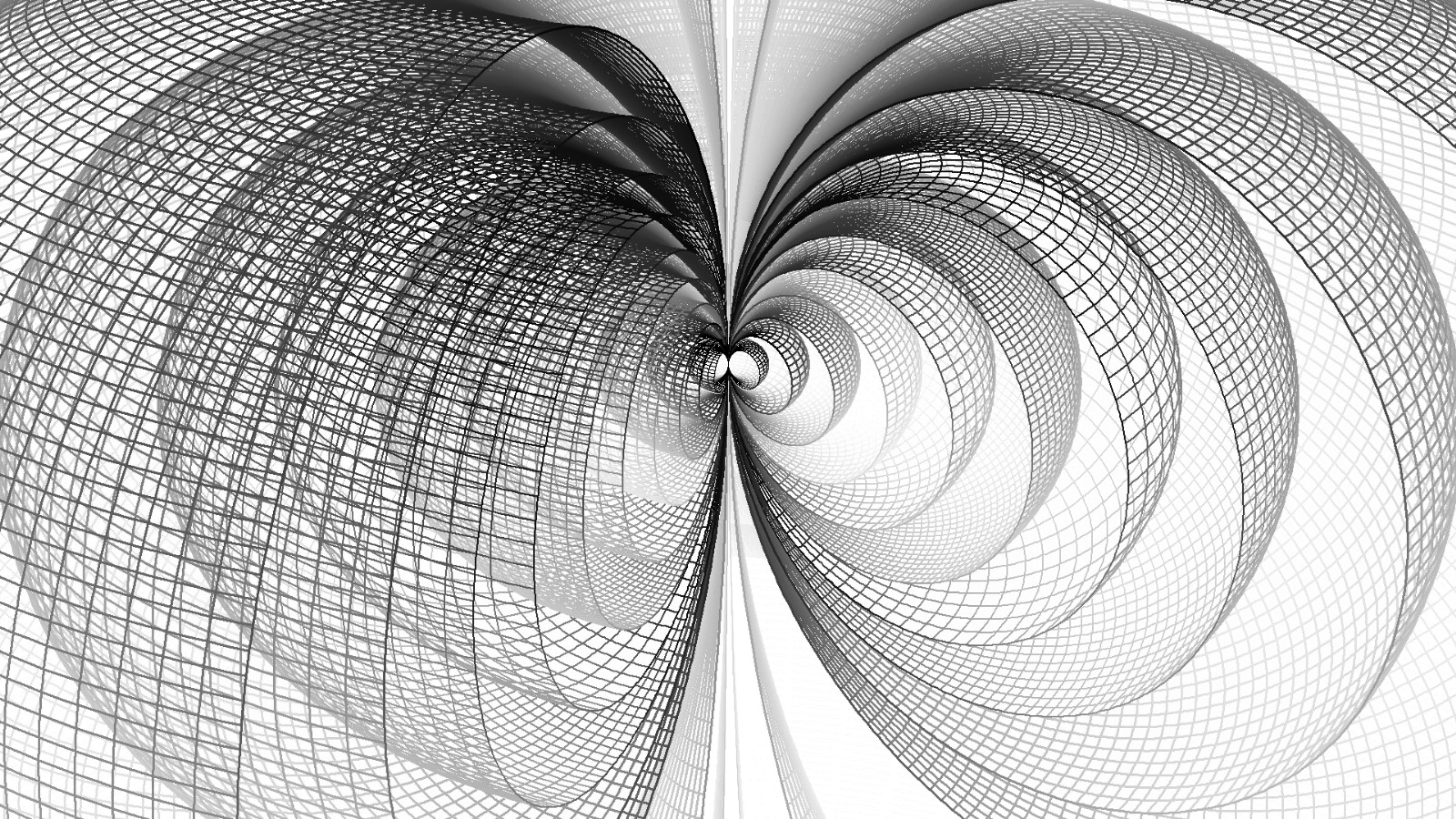
The dynamic, however, as most important intrinsic property of the model, cannot be described properly and comprehensively by static depictions like the above ones. We need moving pictures, animations, simulations for an adequate presentation of dynamic. In this printed publication we have no choice but to provide links to respective webpages, where such animations can be viewed.
 
The linked animation [2] shows a wide section of a single entity in a coarse, simplified depiction of the dynamically uncoiling horn torus coordinate. The ‘unrolling line’ starts parallel to longitudes on a very small horn torus, forms Lissajous figures, when passing rational ratios revolution : rotation, which are shown as ‘resonances’ with short stops during increase of size, and approaches infinite size with parallels to latitudes, without resonances. The animation possibly might lead to the following:
 
Physical interpretation
 
Sharp resonances represent fermions, the sections between them bosons, and the lines without any resonances on big horn tori (ratio << 1) are photons. In this way all kinds of elementary particles are beaded on one thread, within one single coordinate, forming a unique ‘fundamental entity’. What appears to be a ‘particle’ is only the ‘local’ property of the much more comprehensive entity at a specific ‘location’ in the horn torus space. What we describe as a nucleon here, is an electron there, farther away a photon, and simultaneously a completely different ‘particle’ of the same entity elsewhere.
 
Now we combine another or more entities with a singled-out entity by nesting them in their common point S. Place of ‘interaction’, where a horn torus unrolls on a trajectory, always remains this centre. It is the ‘spatial point’, to which any ‘particle’ in the universe is connected as one portion of its individual ‘entity’. All infinite many ‘particles’ of the universe are represented in every ‘spatial point’. Every horn torus shares the common tangent with all other horn tori, when they are nested into one another at their centres. Size of a horn torus symbolises the ‘distance’ to the location, where the associated entity (the dynamic coordinate) converges to size zero. Different ‘spatial points’ differ in the combination of horn torus sizes. All combinations with natural numbers (explication for that see links below) of sizes are possible, forming an infinite-dimensional regular pattern. All paths through this discrete pattern (space) are equally possible, and every horn torus can unroll at a trajectory formed by any other horn torus as interaction at any ‘spatial point’.
 
This space is by its definition a multiverse (due to infinite many paths) with non-local correlations between ‘particles’. A limited neighbourhood of every ‘spatial point’ contains the complete information about one selected universe. With this property one - by the way - immediately recognizes the possibility that big bang has not started out of one tiny spot but rather took place all-over a pre-existing just one-dimensional infinity. Much more information you will find on linked websites.
 
 
* Remarks
 
• In conventional, familiar mathematics and physics, the static horn torus serves as an extension of the well-known Riemann sphere. The existing bijective conformal mapping between both (discovered by me 2018 [6],[7]) distinguishes the horn torus as a member of complex manifolds. So, wherever you apply the Riemann sphere in theoretical physics, you may replace it by the equivalent horn torus and instantaneously gain a lot more properties of the described object!
 
• In retrospect, with a view onto history of science, horn tori could be associated with the ‘ur-alternatives’ by C.F.v. Weizsäcker, but also with spinors, Penrose’s twistors, Lie groups and all related - also current - symmetry considerations.
 
• The (allegoric or analogously figurative) dynamic horn torus model primarily only provides ontologically relevant interpretations. These can be used to justify and substantiate a philosophical worldview that can be assigned to subjective idealism or constructivism, and on the basis of which both axiomatic mathematics and phenomenological physics are regarded as not real (independent of consciousness). As first conclusions, among others, one perhaps could state these:
 
  - There is no empty space in which physics takes place. Complex dynamic processes generate the space, an idle state (statics) does not occur.
  - There are no ‘dimensions’, neither a Euclidean nor non-Euclidean space, nor any vector spaces, not even in the sense of the abstract Hilbert space.
  - Time is not a basic physical quantity, it is included and identifiable in the model, but is redundant and reducible to more fundamental quantities.
  - Without a consciousness that describes them, there are no individual, independent ‘physical objects’, especially no isolated or isolatable particles.
  - All entities, i.e., all ‘particles’ too, are connected (linked, entangled) with all other entities or ‘particles’ of the universe and ‘interact’ with each other.
  - Interacting means being dynamically interlaced, constantly measuring and comparing each other and deterministically integrating into an entirety.
  - The universe is an orderly, mathematically describable network, a regularly structured pattern, the mere structure of an a priori existing mathematics [8].
  - A consciousness uses its special senses to pick out only very small parts of this abstract mathematical pattern from the comprehensive total of processes.
  - All described properties of what is perceived are interpretations by the perceiver, generated by subjective mental processing.
 
• After science for centuries ignored the existence of the geometric figure horn torus completely or neglected its relevance unduly - nearly nobody realized, described and applied the exceptional topology, maximum symmetry, high complexity and creative capabilities of this unique object - it now, in recent years, increasingly appears in publications, mostly in context of particle and quantum physics and in connection to cosmological questions. The subject is developing and promises to stay exciting for a good while.
 
• Postulation as a consistent theory, based on the horn torus model, will be an ambitious project for physicists in the future, after mathematicians and computer programmers will have established methods to handle that dynamic. I'm afraid we need a different, perhaps disruptive, ‘fundamental’ mathematics.
 
• An introductive explanatory video (16 min.) is provided in link [9], also uploaded to YouTube, amongst some entertaining, horn torus related videos.
P.S.: Meanwhile I have deleted my channel and all contents on YouTube, but look here: https://www.artmetic.de/graphics/video/horn-torus-videos.html
 
 
References
 
This is not a scientific publication and there are no external references provided,
all texts and illustrations originate from author’s private websites (2000...2023):
 
  [1]   https://www.horntorus.com/ (provides all information)
  [2]   https://www.horntorus.com/illustration/URLdetail.html
  [3]   https://www.horntorus.com/illustration/increase.html
  [4]   https://www.horntorus.com/text/16_unification.html#txt
  [5]   https://www.horntorus.com/illustration/2nestedhorntori.html
  [6]   https://www.horntorus.com/manifolds/conformal-mapping.pdf
  [7]   https://www.dorntorus.de/d (original texts in German, 2000)
  [8]   https://www.mathematical-universe.com/ (short synopsis)
  [9]   https://www.bighiss.video/ (related explanatory video)
 
 
 
 
The author, Wolfgang W. Däumler, used to be a (theoretical) physicist and a
physician (anaesthesia, emergency medicine and critical care), was/is active in
several more fields (aviation, navigation, architecture, programming, digital art, …),
now retired and lives in Allgäu, South Germany.   W.Daeumler    CV    memoirs 
 

 ↑ top ↑   Horn Torus & Physics   sitemap   part 1