horn torus entity
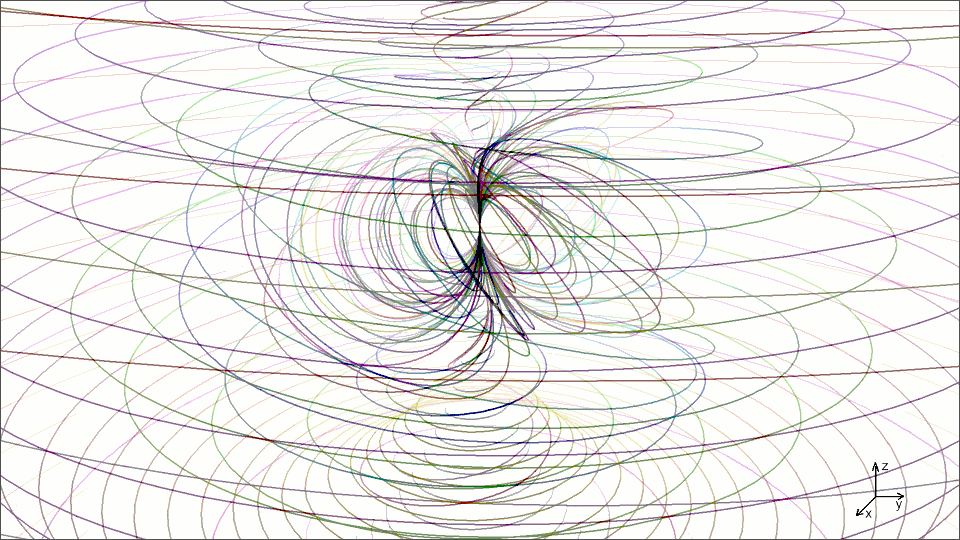
x = r·(1 − cos(ω2/2π))·cosω
y = r·(1 − cos(ω2/2π))· sinω
z = r · sin(ω2/2π)

r = r1·2π / ω for r < r1
ω = 2π when r = r1 *)
ω < 2π doesn't occur !
ω is the toroidal horn
torus angle, r in the graphic above is not to scale
(increments of the single steps
are pretty rough),
depicted is the section
between 4 revolutions per 1 rotation and 100 rotations per
1 revolution
(for explanation click image)
*) r1 signifies the
standard dynamic horn torus, the 'μ-sterious'
mirror between
'infinite outer world', consisting of electrons and photons, and the 'small inner world' of
hadrons and
infinitesimal infinity.
Important note 1 in this context: the widely unknown infinitesimal infinity has the
same cardinality as our known large universe (i.e. nuclei comprise whole universes).
It's worth to think about that!
Note 2, equally imperative: horn torus depictions are allegoric only, their purpose is
to symbolise mathematical particle properties - they don't occur as objects in
our familiar 3-dimensional space.
Dynamic variation of poloidal revolution and toroidal rotation, combined with
alteration of size, can be expressed smartly by complex and hypercomplex numbers
(quaternions, octonions, ...) - but
to describe the whole dynamics as abstract physical processes, including the
interaction of intertwined nested horn tori, seems to be a task, which is
not manageable by conventional
mathematics,
