'complex manifolds' →
Riemann sphere, mapping on horn torus & more (click image or scroll down)
 Complex plane and Riemann sphere are bijective mappings between one another, only infinity has to be treated separately.
Complex plane and Riemann sphere are bijective mappings between one another, only infinity has to be treated separately.
Vyacheslav Puha (Вячеслав Пуха) describes an
additional mapping as stereographic
projection, using the horn torus,
to solve various problems with infinity, especially for treating the mathematically forbidden operation 'division by zero'.
Allowing an analytical alteration, the
horn torus represents the set of complex numbers in a static conformal way too
In my (Wolfgang W. Daeumler's) horn torus model the complex numbers are represented in a totally different way:
the horn tori are dynamic! roughly speaking: real numbers correspond to the angle of rotation (number of rotations)
and imaginary numbers are represented by the length of the unrolling line, which the horn torus travels on the axis
when the bulge of the horn torus executes the revolution around itself and along (!) this centered (symmetry) axis
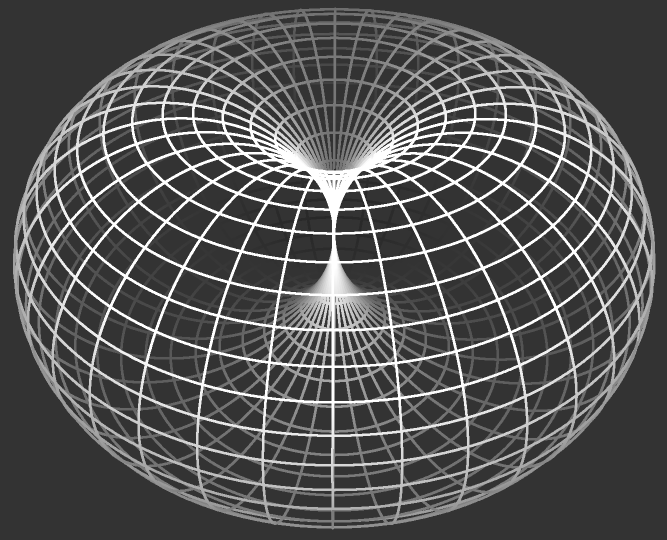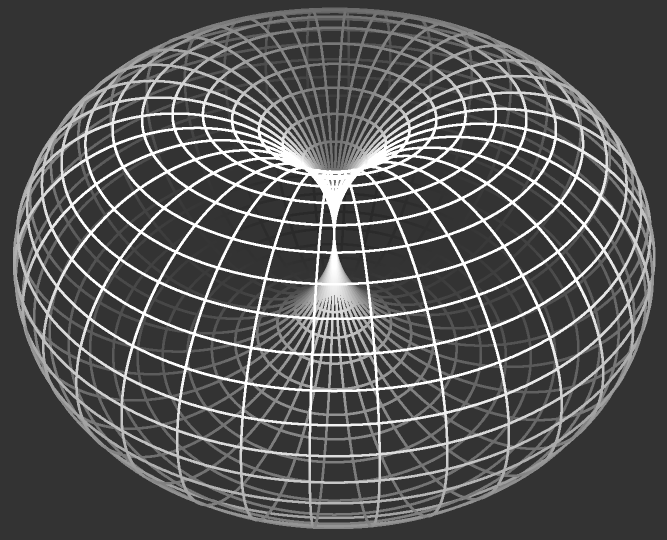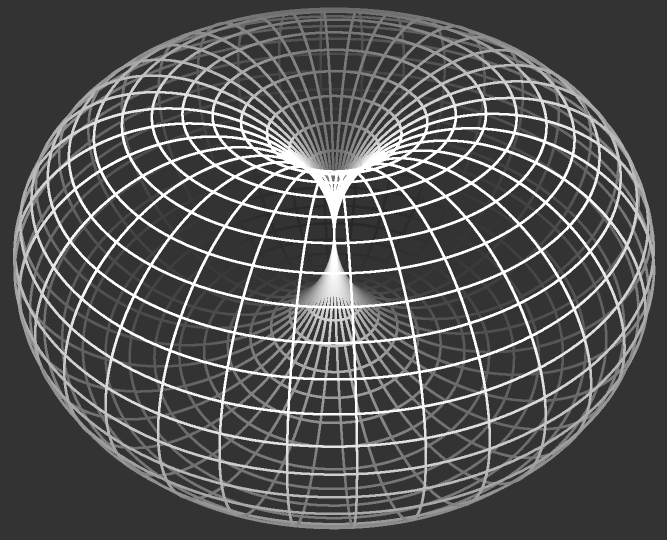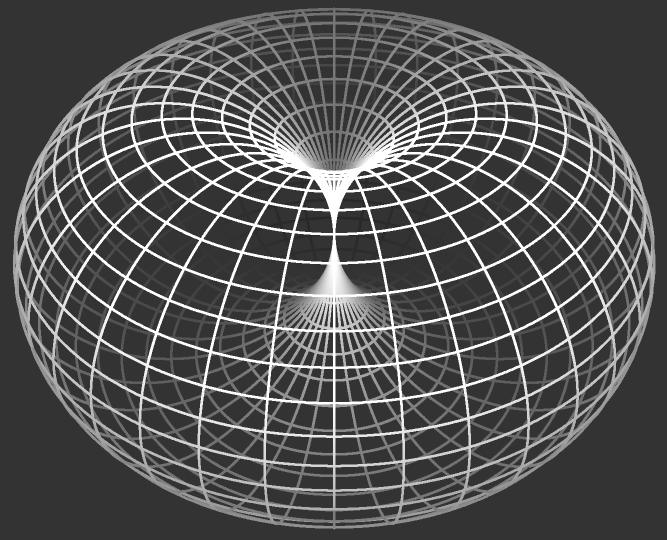
standard dynamic horn torus, version 11 ( spin – / time – )
click an arrow to change direction of turn:
↔
↕
↕↔
(click animation for nested horn tori)
→ construction method
1 ·
2 / back to
frontpage


